785. Is Graph Bipartite?

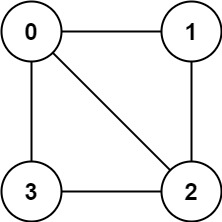
There is an undirected graph with n nodes, where each node is numbered between 0 and n - 1. You are given a 2D array graph, where graph[u] is an array of nodes that node u is adjacent to. More formally, for each v in graph[u], there is an undirected edge between node u and node v. The graph has the following properties:

• There are no self-edges (graph[u] does not contain u).
• There are no parallel edges (graph[u] does not contain duplicate values).
• If v is in graph[u], then u is in graph[v] (the graph is undirected).
• The graph may not be connected, meaning there may be two nodes u and v such that there is no path between them.

A graph is bipartite if the nodes can be partitioned into two independent sets A and B such that every edge in the graph connects a node in set A and a node in set B.

Return true if and only if it is bipartite.

Example 1:

Input: graph = [[1,2,3],[0,2],[0,1,3],[0,2]]
Output: false
Explanation: There is no way to partition the nodes into two independent sets such that every edge connects a node in one and a node in the other.

Example 2:

Input: graph = [[1,3],[0,2],[1,3],[0,2]]
Output: true
Explanation: We can partition the nodes into two sets: {0, 2} and {1, 3}.

Constraints:

• graph.length == n
• 1 <= n <= 100
• 0 <= graph[u].length < n
• 0 <= graph[u][i] <= n - 1
• graph[u] does not contain u.
• All the values of graph[u] are unique.
• If graph[u] contains v, then graph[v] contains u.

Solutions (Rust)

1. Solution

use std::collections::HashSet;

impl Solution {
    pub fn is_bipartite(graph: Vec<Vec<i32>>) -> bool {
        let mut seen = HashSet::new();
        let mut a = HashSet::new();
        let mut b = HashSet::new();

        for i in 0..graph.len() {
            if !seen.contains(&i) {
                let mut insert_a = true;
                let mut nodes0 = vec![i];

                while !nodes0.is_empty() {
                    let mut nodes1 = vec![];

                    for j in nodes0 {
                        seen.insert(j);
                        if insert_a {
                            a.insert(j);
                        } else {
                            b.insert(j);
                        }

                        for &k in &graph[j] {
                            if !seen.contains(&(k as usize)) {
                                nodes1.push(k as usize);
                            }
                        }
                    }

                    insert_a = !insert_a;
                    nodes0 = nodes1;
                }
            }
        }

        a.intersection(&b).count() == 0
    }
}